本文共 3292 字，大约阅读时间需要 10 分钟。

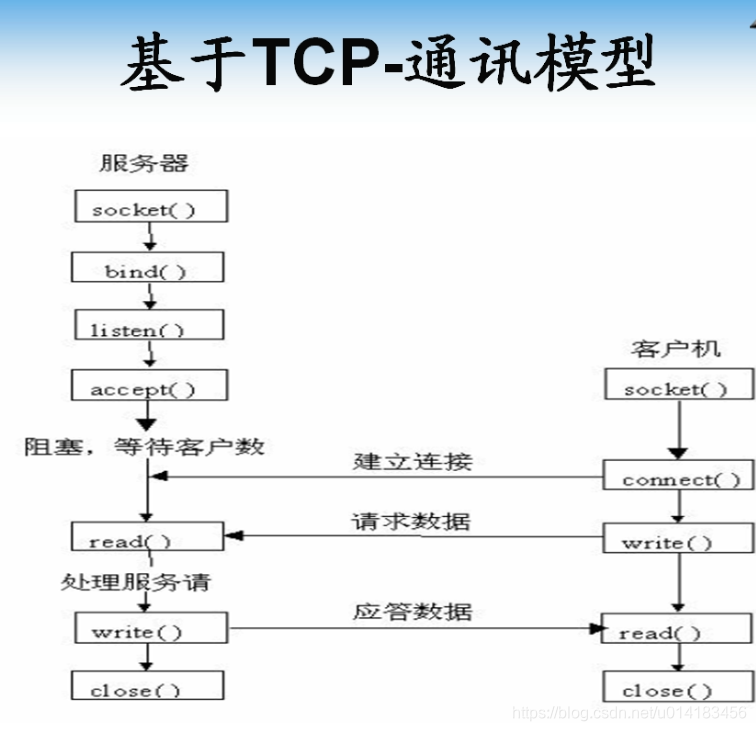
基于TCP-服务器

1. 创建一个socket，用函数socket()
2. 绑定IP地址、端口等信息到socket上，用函数bind()
3. 设置允许的最大连接数，用函数listen()
4. 等待来自客户端的连接请求，用函数accept（）
5. 收发数据，用函数send()和recv()，或者read()和write()
6. 关闭网络连接
   代码

#include 
   
     #include 
    
      #include 
     
       #include 
      
        #include 
       
         #include 
        
          #include 
         
           #include 
          
            #define portnumber 3333int main(int argc, char *argv[]) { int sockfd,new_fd; struct sockaddr_in server_addr; struct sockaddr_in client_addr; int sin_size; int nbytes; char buffer[1024]; /* 服务器端开始建立sockfd描述符 */ if((sockfd=socket(AF_INET,SOCK_STREAM,0))==-1) // AF_INET:IPV4;SOCK_STREAM:TCP { fprintf(stderr,"Socket error:%s\n\a",strerror(errno)); exit(1); } /* 服务器端填充 sockaddr结构 */ bzero(&server_addr,sizeof(struct sockaddr_in)); // 初始化,置0 server_addr.sin_family=AF_INET; // Internet server_addr.sin_addr.s_addr=htonl(INADDR_ANY); // (将本机器上的long数据转化为网络上的long数据)和任何主机通信 //INADDR_ANY 表示可以接收任意IP地址的数据，即绑定到所有的IP //server_addr.sin_addr.s_addr=inet_addr("192.168.1.1"); //用于绑定到一个固定IP,inet_addr用于把数字加格式的ip转化为整形ip server_addr.sin_port=htons(portnumber); // (将本机器上的short数据转化为网络上的short数据)端口号 /* 捆绑sockfd描述符到IP地址 */ if(bind(sockfd,(struct sockaddr *)(&server_addr),sizeof(struct sockaddr))==-1) { fprintf(stderr,"Bind error:%s\n\a",strerror(errno)); exit(1); } /* 设置允许连接的最大客户端数 */ if(listen(sockfd,5)==-1) { fprintf(stderr,"Listen error:%s\n\a",strerror(errno)); exit(1); } while(1) { /* 服务器阻塞,直到客户程序建立连接 */ sin_size=sizeof(struct sockaddr_in); if((new_fd=accept(sockfd,(struct sockaddr *)(&client_addr),&sin_size))==-1) { fprintf(stderr,"Accept error:%s\n\a",strerror(errno)); exit(1); } fprintf(stderr,"Server get connection from %s\n",inet_ntoa(client_addr.sin_addr)); // 将网络地址转换成.字符串 if((nbytes=read(new_fd,buffer,1024))==-1) { fprintf(stderr,"Read Error:%s\n",strerror(errno)); exit(1); } buffer[nbytes]='\0'; printf("Server received %s\n",buffer); /* 这个通讯已经结束 */ close(new_fd); /* 循环下一个 */ } /* 结束通讯 */ close(sockfd); exit(0); }
          
         
        
       
      
     
    
   

基于TCP-客户端

1. 创建一个socket，用函数socket()
2. 设置要连接的服务器的IP地址和端口等属性
3. 连接服务器，用函数connect()
4. 收发数据，用函数send()和recv()，或者read()和write()
5. 关闭网络连接

#include 
   
     #include 
    
      #include 
     
       #include 
      
        #include 
       
         #include 
        
          #include 
         
           #include 
          
            #define portnumber 3333int main(int argc, char *argv[]) { int sockfd; char buffer[1024]; struct sockaddr_in server_addr; struct hostent *host; /* 使用hostname查询host 名字 */ if(argc!=2) { fprintf(stderr,"Usage:%s hostname \a\n",argv[0]); exit(1); } if((host=gethostbyname(argv[1]))==NULL) { fprintf(stderr,"Gethostname error\n"); exit(1); } /* 客户程序开始建立 sockfd描述符 */ if((sockfd=socket(AF_INET,SOCK_STREAM,0))==-1) // AF_INET:Internet;SOCK_STREAM:TCP { fprintf(stderr,"Socket Error:%s\a\n",strerror(errno)); exit(1); } /* 客户程序填充服务端的资料 */ bzero(&server_addr,sizeof(server_addr)); // 初始化,置0 server_addr.sin_family=AF_INET; // IPV4 server_addr.sin_port=htons(portnumber); // (将本机器上的short数据转化为网络上的short数据)端口号 server_addr.sin_addr=*((struct in_addr *)host->h_addr); // IP地址 /* 客户程序发起连接请求 */ if(connect(sockfd,(struct sockaddr *)(&server_addr),sizeof(struct sockaddr))==-1) { fprintf(stderr,"Connect Error:%s\a\n",strerror(errno)); exit(1); } /* 连接成功了 */ printf("Please input char:\n"); /* 发送数据 */ fgets(buffer,1024,stdin); write(sockfd,buffer,strlen(buffer)); /* 结束通讯 */ close(sockfd); exit(0); }
          
         
        
       
      
     
    
   

转载地址：http://yebzz.baihongyu.com/